Homework 9 (Solutions)

Homework Assignment 9

Intermediate Microeconomics (Econ 100A)
Kristian López Vargas
UCSC

Instructions:

You will scan your homework into a single PDF file to be upload.
Only legible assignments will be graded.
Late assignments will not be accepted.

Only two randomly-chosen questions will be graded.

Question 1:

Suppose two firms, Genera Pharma and Futura Pharma, are the only two producers of a specific drug. Genera and Futura have the same formula and sell the drug for the same price, but they are considering whether or not to spend money on an advertising campaign. Each firm can either buy the advertising campaign or not buy it and the following table shows profits for four different scenarios.

		Futura
		AD	No AD
Genera	AD	(4,4)	(12,0)
Genera	No AD	(0,12)	(8,8)

What is the scenario that maximizes the sum of profits of the two companies?
Is there a dominant strategy for each firm?
What is the pure strategy Nash Equilibrium?
Denote the probability of choosing $AD$ for Futura as $f$ and for Genera as $g$. Find the best response function for Futura, $b_f(g)$, and Genera, $b_g(f)$.

Solutions:

The best collective outcome is when neither firm buys advertising $($ ${$}$16 million combined payout > ${$}$12 m > ${$}$8m $)$
Advertising is the dominant strategy for both firms.
The pure strategy Nash equilibrium is for both firms to advertise.
Then Futura's payoff is: $$\begin{align} &= 4fg + 12f(1-g) + 0(1-f)g + 8(1-f)(1-g) \\ &= 4fg + 12f - 12fg + 8 - 8f - 8g + 8fg \\ &= 4f + 8 - 8g\\ \end{align}$$ It is maximized when $f^{*}=1$ regardless of the choise of the opponent. Hence, $b_f(g) = 1$. Genera's payoff is: $$\begin{align} &= 4fg + 0f(1-g) + 10(1-f)g + 8(1-f)(1-g) \\ &= 4fg + 12g - 12fg + 8 - 8f - 8g + 8fg \\ &= 4g + 8 - 8f \\ \end{align}$$ It is maximized when $g^{*}=1$ regardless of the choise of the opponent. Hence, $b_g(f) = 1$

Question 2:

Below table shows the probability of winning for Roger Federer and Rafael Nadal for each of two groundstrokes in tennis: down the line (DL) and crosscourt (CC).

		Rafael Nadal
		DL	CC
Roger Federer	DL	(5,5)	(8,2)
Roger Federer	CC	(9,1)	(2,8)

Find the pure strategy NE if any.
Find the mixed strategy NE if any.
Find the probability of winning of Federer and Nadal, respectively.

Solutions:

There's no pure strategy NE.
Federer solves for the value of p that equates Nadal’s payoff from positioning himself for DL or CC: $$\begin{align} 5p+(1‐p) &= 2p+8(1‐p) \\ 5p+1‐p &= 2p+8‐8p \\ 4p+1 &= 8‐6p \\ 10p &= 7 \\ p &= 7/10 = .70. \end{align}$$ Nadal solves for the value of q that equates Federer's payoff from positioning herself for DL or CC: $$\begin{align} 5q+8(1‐q) &= 9q+2(1‐q) \\ 5q+8‐8q &= 9q+2‐2q \\ 8‐3q &= 7q+2 \\ 6 &= 10q \\ q &= 6/10 = .60. \end{align}$$
Nadal's payoff from DL is $DL=5(.70)+1(.30)=3.8$ and the payoff from CC is $CC=2(.70)+8(.30)=3.8$. Following the same logic, Federer's payoff from DL and CC is the same, we will get Federer's payoff is 6.2.

The question is asking about the probability of winning, actually the payoff is the probability of winning in this context if we read the first sentence of the question. It will be easier to understand it if we say the unit for all the numbers in the payoff matrix is 0.1 (e.g. If both of them choose DL, the probability of winning(payoff) for them are (0.5, 0.5).) Then the answer for part 1 and part 2 are the same, the answer for part 3 is: the probability of winning of Federer is 0.62, the probability of winning of Nadal is 0.38.

Question 3: Coordination Game

Consider the South Korea - North Korea arms race in which each country could build nuclear missiles or refrain from building them. The payoffs are shown in table below.

		North Korea
		Build	Refrain
South Korea	Build	(6,6)	(5,1)
South Korea	Refrain	(3,8)	(12,12)

What are the dominant strategies for each country if any?
What are the pure strategy NE?
Denote $n$ as the probability NK will build nuclear missles and $s$ as the probability SK will build nuclear missles. Find the best response function for each country, $b_s(n)$ and $b_n(s)$.
Find the mixed strategy NE in light of the answer in part 3

Solutions:

There is no dominant strategy for either country.
$(Build, Build)$ and $(Refrain, Refrain)$ are the pure strategy equilibria.
NK's payoff: $$ \begin{align} &= 6sn + 8(1-s)n+1(1-n)s+12(1-s)(1-n)\\ &= 6sn + 8n - 8sn + s - sn + 12 - 12n - 12s + 12sn\\ &= 9sn - 11s + 12 - 4n \\ &= n(9s-4) + 12 - 11s \end{align} $$ Hence NK's best response is, $$ b_n(s) =\begin{cases} 0 & if\ s < \frac{4}{9} \\ \in\left[0,1\right] & if\ s = \frac{4}{9} \\ 1& if\ s > \frac{4}{9} \\ \end{cases} $$ SK's payoff: $$ \begin{align} &= 6sn + 5(1-n)s+3(1-s)(n)+12(1-s)(1-n)\\ &= 6sn + 5s - 5sn + 3n - 3sn + 12 - 12n - 12s + 12sn\\ &= 10sn - 7s - 9n + 12\\ &= s(10n - 7)-9n + 12 \end{align} $$ Hence SK's best response is, $$ b_s(n) =\begin{cases} 0 & if\ n < \frac{7}{10} \\ \in\left[0,1\right] & if\ n = \frac{7}{10} \\ 1& if\ n > \frac{7}{10} \\ \end{cases} $$
$(n=\frac{7}{10}, s=\frac{4}{9})$ is the mixed strategy equilibrium.

Question 4: Centipede game

The centipede game, first introduced by Robert Rosenthal in 1981, is an extensive form game in which two players take turns choosing either to take a slightly larger share of an increasing pot, or to pass the pot to the other player. In other words, player 1 chooses between D $($Down$)$ and A $($Across$)$, where D is pocketing the pot and A is passing the pot to the player 2. Similarly, player 2 chooses between $D$ and $A$.

The payoffs are arranged so that if one passes the pot to one's opponent and the opponent takes the pot on the next round, one receives slightly less than if one had taken the pot on this round.

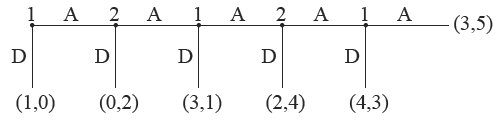
Find the subgame perfect Nash Equilibrium using backward induction.

Solutions:

For backward induction, we start from the last node at which player 1 decides between $D$ which gives $(4,3)$ and $A$ which gives $(3,5)$. Since $4>3$, the player 1 will choose $D$. Now on the second node from the last, knowing player 1 will choose $D$, player 2 chooses between $(2,4)$ and $(4,3)$. Since $4>3$, player 2 chooses $D$. Similarly, on the third node from the last, player 1 chooses $D$, and finally on the very first node, player 1 chooses $D$.

To write down the equilibrium of strategies, strategies have to be specified at every node of decisions. There are three nodes $($1st, 3rd, 5th$)$ for player 1 and two nodes $($2nd, 4th$)$ for player 2. In a long version, $($Player 1 plays $D$ in her 1st node, Player 1 plays $D$ in her 2nd node, Player 1 plays $D$ in her 3rd node; Player 2 plays $D$ in her 1st node, Player 2 plays $D$ in her 2nd node$)$ is the subgame perfect NE. In a short version, $(DDD; DD)$ is the subgame perfect NE.

Question 5: Ultimatum Game

The ultimatum game is a game in economic experiments. The first player $($the proposer$)$ receives a sum of money and proposes a fair proposal $($F - 5;5$)$ or unfair proposal $($U - 8;2$)$. The second player $($the responder$)$ chooses to either accept $($A$)$ or reject $($R$)$ this proposal. If the second player accepts, the money is split according to the proposal. If the second player rejects, neither player receives any money.

Find the subgame perfect Nash Equilibrium using backward induction.

Solutions:

Given the fair proposal, the second player choose to accept the proposal because $5 > 2$. Given the unfair proposal, the second player chooses to accept the proposal because $2 > 0$. Knowing this, the first palyer will choose $U$ over $F$ because $8>5$.

To write down the equilibrium of strategies, strategies have to be specified at every node of decisions. There is one node for player 1 and two nodes for player 2. In a long version, $($Player 1 plays $U$; Player 2 plays $A$ if player 1 plays $F$, Player 2 plays $A$ if player 1 plays $U$ $)$ is the subgame perfect NE. In a short version, $(U; AA)$ is the subgame perfect NE.

In Reality, according to this, "When carried out between members of a shared social group $($e.g., a village, a tribe, a nation, humanity$)$ people offer "fair" $($i.e., 50:50$)$ splits, and offers of less than 30% are often rejected."